Measure CO2 – Part 3 – The Source & Detector

It’s hard to talk of one without considering the other. A proper absorption wavelength of the laser needs to be considered as does a corresponding detector. The species defines the possible wavelengths, the detector defines which wavelengths can be captured, and a feasible laser needs to generate that wavelength.

The Source

The quantum theory of electromagnetics assumes that all EM radiation consists of photons as elementary particles. These quantum effects also allow photons to be created by causes such as the transition of electrons to lower atomic energy states and black-body radiation. The energy of an individual photon is discrete but dependent on photon frequency per Planck’s equation:

$\mathcal{E}_\gamma \; = \; \mathpzc{h} \, \text{c} \, \nu$

where $\mathpzc{h}$ is Planck’s Constant, c is photon velocity (speed-of-light in air), and $\nu$ is the photon frequency.

It is usually convenient to refer to photon wavelength where:

$\mathcal{E}_\gamma \; = \; \frac{\, \mathpzc{h} \, \text{c} \, }{\lambda}$

where $\lambda$ is the photon wavelength.

For this discussion, wavelengths on the order of 1500 nm are of interest; this represents a photon frequency of 200 THz. Visible light has wavelengths in the range of 380 nm (violet) to 750 nm (far red) – extreme human range of visibility ranges from 310 – 1050 nm.

Geometry
The actual beam does not project “straight” – the laser projects a Gaussian beam which is radially symmetrical distribution whose electric field variation is given by the following equation :

$\mathcal{E}_G \; = \; \mathcal{E}_o \; exp \left(\text{-} \frac{r^2}{\, w^2 \, } \right)$

where r is defined as the radial distance from the center of the beam, and w is the radius at which the amplitude is 1/e of its value on the axis.

A Gaussian source distribution remains Gaussian at every point along its path of propagation through the optical system. This makes it particularly easy to visualize the distribution of the fields at any point in the optical system. The intensity is also Gaussian :

$\Phi \; = \; \eta \, \mathcal{E}_o \, \mathcal{E}_o^* \, exp \left(\text{-} \frac{\, 2 \, r^2 \,}{\, w^2 \, } \right)$

where $^*$ denotes the complex conjugate.

Beam Intensity

The intensity across the axis appears as:

The laser beam divergence is assumed to be 0.15 mrad resulting in a beam diameter of 3 m at the reflecting surface (the Earth surface). The intensity of the beam over the circular illumination area is of the form:

The measurement platform is approximately stationary with respect to measurement time therefore the source and reflected beams have an angle between them that allows tan Θ ~ Θ where Θ → 0.

Assume an optical power of 1 W is evenly distributed across the aperture which has a diameter of 1 cm (while this is not strictly the case, it may be assumed a reasonable approximation for the distances under discussion. The interest is an approximation of the intensity which impinges the reflecting surface)

Optical intensity is power per area; the aperture area is found from:

$\text{area} \; = \; \pi \, 0.005^2 \; = \; 78.54 \times 10^{-6} \; m^2$

The intensity – or rather, irradiance (although both terms may be used interchangeably in this situation) – at the aperture … 1 W power per aperture area (W/m $^2$ ) is:

$\Phi_o \; = \; \frac{\, \text{power} \, }{\text{area}} \; = \; \frac{1}{\, 78.54 \times 10^{-6} \, } \; = \; 12,732.4 \; W/m^2$

Expressed in terms of number of photons where W = joules/sec. The energy of each photon is dependent on the wavelength. A laser bam is a coherent flux of photons having identical energy – the energy of a photon with wavelength of 1571 nm (wavenumber = 6365.37 cm $^{-1}$ ) is:

$\mathcal{E}_\gamma \; = \; \frac{\, \mathpzc{h} \, \text{c} \, }{\lambda} \; = \; \frac{\, 6.6261e\text{-34} \times 3001e\text{6} \, }{1571e\text{-9}} \; = \; 126.4e\text{-21} \; \text{J}$

which leads to the number of photons per J of energy:

$\text{n}_\gamma \; = \; \frac{1.000}{\, 126.4e\text{-21} \, } \, \frac{\text{J/sec}}{\, \text{J/}\gamma \, } \; = \; 7.91e\text{18} \; \gamma/\text{sec}$

Transverse Electromagnetic Propagation
A laser is a directed monochromatic beam; the energy is not isotropic, but is rather more of the form of a transverse electromagnetic field along a transmission line which, for the electric field component, is defined as:

$\mathpzc{E} \; = \; \mathpzc{E}_o \, exp[\, \text{-}\jmath \, \mathpzc{k} \cdot \mathpzc{r} \, ]$

where $\mathpzc{E}$ is the received electric field characterized by the free-space wavenumber k and position vector r.

A wave propagating in the z-direction through a finite media which is then reflected back along the z-axis may be expressed:

$\mathpzc{E} \; = \; \mathpzc{E}_o^+ \, exp[\, \text{-}\jmath \, \gamma \, z \, ] \; + \; \mathbb{R} \; \mathpzc{E}_o^- \, exp[\, \text{+}\jmath \, \gamma \, z \, ]$

where $\gamma$ is the material property coefficient: $\gamma \; = \; \alpha \, + \, \jmath \, \beta$ which leads to a form of:

$\begin{displaymath} \begin{align} \mathpzc{E}_r \; &= \; \mathpzc{E}_o \; exp[\, \text{-}\alpha \, z \,] \; exp[\, \text{-}\jmath \, \beta \, z \,] \; exp[\, \text{-}\jmath \, \omega \, t \,] \\ \\ &= \; \mathpzc{E}_o \, exp[\, \text{-}z \, (\alpha + \jmath \beta) - \jmath \, \omega \, t \,] \end{align} \end{displaymath}$

Term α is real and represents field attenuation. As distance z increases, the term tends to zero. “Skin depth” is defined as the distance in which the magnitude has decreased by a factor of $e^{\text{-}1}$ related to the initial magnitude.

Term β represents a wave varying sinusoidally with:

$exp[\, \text{-}\jmath \, \beta \, z \,] \; \; \Rightarrow \; \; cos \, \beta \, z \; - \; \jmath \, sin \, \beta \, z$

For this discussion, β may be assumed zero.

Finally, $exp[\, \text{-}\jmath \, \omega \, t \,]$ represents a wave varying sinusoidally with t at frequency ω. However, for transmission purposes, the photon frequency “doesn’t exist” and the continuous flux allows $\omega$ to be zero. If the beam source were sinusoidally modulated, $\omega$ would be the frequency of modulation (atmospheric effects will modulate the signal, but exponentially).

So I end up with the propagation expression:

$\mathpzc{E}_r \; &= \; \mathpzc{E}_o \; exp[\, \text{-}\alpha \, z \,]$

Pictorially, this appears as:

The frequency of photon vibration is so high related to the optical distance, that the beam may be considered “solid” with amplitude represented by the BLU trace. If the beam were modulated, the signal would be as indicated by the GRN and MAG traces (if this illustration is to scale with an altitude of 10 km, the wavelength of the sinusoids would be about 1.25 km; the frequency about 240 kHz)

Beam Geometry Revisited

A cylindrical volume is often assumed due to the length of the travel path with respect to the beam diameter. Density being a measure of particles per volume, it may prove beneficial to consider the actual optical volume – even if idealized. The actual (ideal) beam volume based on far-field geometry can be modelled as shown:

The illustration is to scale – radial scale is multiplied by 1000; the beam radius at the reflecting surface is (z θ) where θ is the beam divergence angle: 0.2 mrad for this illustration. The receiving lens diameter is 0.4 m (~ 16”)

The cylindrical area is based on a uniform cross-section defined by the beam area at the ideal reflecting surface. The GRN-ish cone represents the beam when transmitted by a laser with opening less than 1 cm over a distance of 10,000 m with beam divergence θ. The RED-ish truncated cone represents a telescope with a field-of-view matching the beam area at the reflecting surface and gathered by a lens of 0.4 m diameter. The PUR-ish cylinder is the representation using the surface beam diamter as the volume diameter.

Consider that much analysis has been based on V_T = V_R = cylinder. This implies that:

$\text{V}_{RX} \; = \; \text{V}_{TX} \; = \; \text{V}_{cyl} \; = \; \pi \, \text{h r}_1^2$

so that the transmit and receive volumes are identical.

A more accurate representation takes into account the truncated cone nature of the volume:

$\begin{displaymath} \begin{align} \text{V}_{tot} \; &= \; \text{V}_{TX} \, + \, \text{V}_{RX} \\ \\ &\text{V}_{TX} \; = \; \frac{\pi}{\, 3 \, } \, \text{h r}_1^2 \\ \\ &\text{V}_{RX} \; = \; \frac{\pi}{\, 3 \, } \, \text{h} \left( \, \text{r}_1^2 \, + \, \text{r}_1 \, \text{r}_1 \, + \, \text{r}_2^2 \, \right) \\ \\ \text{V}_{tot} \; &= \; \frac{\pi}{\, 3 \, } \, \text{h} \left( \, 2 \, \text{r}_1^2 \, + \, \text{r}_1 \, \text{r}_1 \, + \, \text{r}_2^2 \, \right) \; \; \neq \; \; \pi \, \text{h r}_1^2 \end{align} \end{displaymath}$

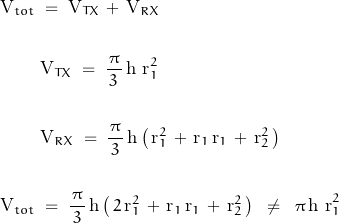
The absorption coefficient α is derived from the product of the number of absorbers and the effective absorber cross-sectional area. By assuming the species absorption cross-section is constant, the optical depth is more properly expressed as:

$\begin{displaymath} \begin{align} \vartheta \; &= \; \int\limits_0^\mathcal{H} \alpha \, \sigma \text{d}z \; \; \Rightarrow \; \; \int\limits_0^\mathcal{H} \alpha \, [ \, \sigma_T(z) \, + \, \sigma_R(z) \, ] \, \text{d}z \\ \\ &= \; \int\limits_0^\mathcal{H} \alpha \, [ \, \sigma_T(z) \, ] \, \text{d}z \; + \; \int\limits_0^\mathcal{H} \alpha \, [ \, \sigma_R(z) \, ] \, \text{d}z \\ \\ &= \; \alpha_T \, \frac{\, \pi \, }{3} \, \frac{ \, \mathcal{H} \, }{2} \, \left( \, \theta \, \mathcal{H} \, \right)^2 \; + \; \alpha_R \, \frac{\, \pi \, }{3} \, \frac{ \, \mathcal{H} \, }{2} \, \left[ \, \left( \, \theta \, \mathcal{H} \, \right)^2 \, + \, \theta \, z \, \text{r}_2 \, + \, \text{r}_2^2 \, \right] \end{align} \end{displaymath}$

where σ represents the beam cross-sectional area as a function of distance z. In this calculation, parameter r $_2$ represents the fixed radius of the receiving telescope lens.

Assuming a uniform planar distribution, the quantity of CO2 is necessarily different in the transmit and receive optical depth paths due to differing volumes.

The return path volume is greater than the transmit volume as a function of receiving lens diameter.

Consider a cylindrical volume formed of a cross-section area equal to the beam diameter at the reflecting surface. The optical path volume for θ = 0.2 mrad would be:

$\text{V}_{cyl} \; = \; \pi \, z \, r^2 \; = \; 125,664 \; \text{m}^3$

where r is a fixed quantity of some dimension: r = ( θ $\mathcal{H}$ ) ⇒ 2 m in this example.

Beam divergence angle will have a significant influence on the measurement. Using the expanded representation of the optical volume as a cone during transmission (TX) and a truncated cone on the receive path (RX) gives results of:

$\begin{displaymath} \begin{align} \text{V}_{TX} \; = \; \frac{\, \pi \, }{3} \, \mathcal{H} \, \left( \, \theta \, \mathcal{H} \, \right)^2 \; = \; 41,888 \; \text{m}^3 \\ \\ \text{V}_{RX} \; = \; \frac{\, \pi \, }{3} \, \frac{ \, \mathcal{H} \, }{2} \, \left[ \, \left( \, \theta \, \mathcal{H} \, \right)^2 \, + \, \theta \, z \, \text{r}_2 \, + \, \text{r}_2^2 \, \right] \; = \; 47,778 \; \text{m}^3 \end{align} \end{displaymath}$

where a telescope lens diameter of 0.5 m (~ 20″) has been assumed. The RX volume is 14% larger than the TX volume.

The total volume of the cylinder approximation is 2 x 125,664 m $^3$ = 251,328 m $^3$ .
The total volume of the conical approximation is 41,888 + 47,778) = 89,666 m $^3$ .

The conical approximation is 64% smaller than the cylindrical approximation.

The calculation of CO2 is highly dependent on volume $^\textbf{\normalsize 2}$ .

The quantity measured is number of attenuation elements. The differential method of measure compares a measure of all attenuation elements within an optical volume to a measure of all attenuation elements except CO2 absorption in order to derive a value for CO2 absorption.

Not only are two accurate measures of the number of attenuation elements necessary, but a measure of two path volumes of better accuracy is necessary.

$\frac{\; \text{number of all attenuation n} \, - \, \text{number of all except CO}_2 \; }{\text{volume of transit path} \, + \, \text{volume of receive path}} \; = \; \frac{ \, \text{number of CO}_2 \, }{\text{total volume}} \; = \; \text{CO}_2 \; \text{density}$

The difference between the TX and RX path volumes is more significant than expected. The sensitivity of the measurement to the absolute volume of the optical path makes geometrical knowledge paramount.

Even in this idealized “best-case” illustration, the sensitivity to the volume of the optical path is high.

Consider a system with divergence θ ~ 0.17E-3 rad and lens diameter r $_2$ ~ 0.1 m (8”) lens. For a fixed distance z = 10 km, the volume sensitivity with respect to beam divergence angle and lens radius is:

$\mathcal{S}_V^\theta \; = \; \frac{\theta}{\, \text{V} \, } \, \left. \frac{ \, \partial \text{V} \, }{\partial \theta}\right|_{\text{z = 10 km}} \; \; \Rightarrow \; \; \left. 1.94 \right|_{{r}_2\text{=5 cm}}$

$\mathcal{S}_V^{r_2} \; = \; \frac{r_2}{\, \text{V} \, } \, \left. \frac{ \, \partial \text{V} \, }{\partial r_2}\right|_{\text{z = 10 km}} \; \; \Rightarrow \; \; \left. 0.062 \right|_{\theta \text{=0.17 mrad}}$

The volume is much more sensitive to changes in dispersion angle than the lens radius.

Wavelength Selection

Here’s where the rubber meets the road … the photon wavelength must be at an absorption frequency of the species desired. The wavelength selected also has to be detectable – an appropriate photodetector must be available. For this experiment, I’ll use a photodetector with suitable responsivity in the near-infrared band between 1300 and 1650 nm.

Carbon dioxide (CO2) and methane (CH4) are two gases of strong interest. Using SpectralCalc, I see the spectra appear as:

where RED is methane; BLK is CO2. This, however, is a log scale – the instrument measures a linear scale (although log processing is a possibility and should be considered considering the signal is of a log/exp nature).

The following shows the response on a linear scale. C2 is quite prominent in three regions … but I haven’t examined other atmospheric constituents such as water vapour. Not going to right now either – I could spend an article discussing wavelength selection.

For this experiment, I’ll pick a wavelength in the neighbourhood of 1570 nm. The exact wavelength will be more precise than this. The photodetector is not capable of the desired discrimination; to prevent overlap, an optical filter will be used – these can provide bandpass filtering in the neighbourhood of ±20 pm.

The photon/molecular interaction will result in a linear loss of beam intensity.

Optical Path

In a lossless optical path, the power impinging the reflecting surface will be equal to the power transmitted. The intensity however is inversely proportional to the radii of the beam.

$\begin{displaymath} \begin{align} \Phi \text{\footnotesize (z)} \; &= \; \Phi_o \, \frac{\text{area}\text{\footnotesize (0)}}{\, \text{area}\text{\footnotesize (z)} \,} \, \text{W/m}^2 \\ \\ &= \; 12,732.4 \, \frac{\pi \, 0.005^2}{\, \pi \, 1.5^2 \,} \; = \; 141.47 \; \text{mW} \end{align} \end{displaymath}$

In this lossless situation, the number of photons doesn’t change; the concentration per unit area changes.

The scattering cross-section for molecules in infrared bands is much smaller than the absorption cross-section such that the loss of photons is dominated by absorption; scattering losses can often be neglected. However, ice crystals and water droplets are of similar size as the IR wavelengths; while losses through absorption tends to still dominate, scattering losses may become more significant due to increased humidity.

Scattering losses int he IR band between 1000 and 2000 nm has been estimated as approximately 100 $e\text{-}5$ /m. Assuming no absorption, the intensity at the reflecting surface may now be estimated as:

$\Phi \text{\footnotesize (10km)} \; &= \; \Phi_o \, exp[\, \text{-}10,000 \times 100\text{e-}6 \, ] \; = \; 12,732.4 \times 0.3679 \; = \; 4684 \; \text{W/m}^2$

Assuming perfect reflection and detection, the intensity at the receiver would be 1723.14 W/m $^2$ .

The optical path is subject to both variations in the “real” atmosphere as well as having a dependency on the atmospheric model used. This is discussed elsewhere … as are the variations and effects of the reflecting surface.

The numbers presented here represent “best-case” estimates; for example, if nothing else changes and the effective reflection coefficient is 0.1, the received intensity would be 172.3 W/m $^2$ . Given that absorption losses are dominant, the received signal can be expected to be much less than this value.

The Photodiode

Photons are converted to electrons via the photoelectric effect. In a manner of speaking, this is similar to molecular absorption.

For now, assume a 1:1 correspondence between photon energy and charge creation with 100% efficiency in the photodiode having a transductance factor of hÂP = i where h is dimensionless conversion efficiency, Â is responsivity in A/W, P is optical power (energy in units of joules per photon times intensity in photons per second), and i is photodiode output current (a “typical” value of Â might be on the order of 10A/W)

The detector will require a minimum optical power to provide a usable measurement; it also has a maximum limit of optical power beyond which the detector will possibly be destroyed. While there are potential techniques to extract certain types of signals from below the noise floor (at a cost of increased complexity), the upper limit is defined by the risk of physical damage. These limits define the detector dynamic range; the laser transmit power and expected intensity losses need to be matched with a detector to maximize a measurement dynamic range.

All else is secondary.

6666666666666666666666
If an electron within a material (such as a semiconductor lattice) absorbs the energy of a photon and acquires more energy than the electron binding energy of the material, it breaks free of the lattice structure and forms a free charge carrier. If the photon energy is too low, the electron remains bonded to the lattice.

The release of an electron is dependent on the energy of an individual photon via the optical wavelength rather than intensity – an increase of intensity of low-energy photons simply increases the number of low-energy photons; a change in intensity does not increase the energy of any single photon. The creation of emitted electrons depends only on the energy of an individual photons. This energy transfer occurs between the incident photon and the outermost valence electrons.

Electrons will absorb energy from photons but in an all-or-nothing manner: all of the energy from one photon must be absorbed and used to liberate one electron from atomic binding. If not, the energy is re-emitted. If the photon energy is absorbed, some of the energy liberates the electron from the atom, and the rest contributes to the electron’s kinetic energy as a free particle.

The detection of an optical beam is a fundamentally continuous time process for optical intensities of interest. Optical intensity (power per unit area in J/s/m $^2$ ) and optical power (energy per second in J/s) are numerically identical quantities if the beam intensity is normalized to the detector area. A photodiode converts photons to charge-carrying electrons. (Electrical current has units of charge/sec). Measuring intensity with a photodiode implies measuring charge over some period of time.

The photodetector used as an example is sensitive to optical wavelengths in the vicinity of 1550 nm. As such, each photon has energy:

$\mathcal{E}_\gamma \; = \; \frac{\, h \, \text{c} \, }{\lambda} \, \frac{\, \text{J} \cdot \text{s} \times \text{m/s} \, }{\text{m}} \; \; \Rightarrow \; \; \mathcal{E}_\gamma \; = \; \frac{\, \oldstylenums{6.626}e\text{-34} \times \oldstylenums{300}e\text{6} \, }{\oldstylenums{1550}e\text{-9}} \; = \; \oldstylenums{128}e\text{-21}} \; \text{J}$

Power is energy per sec: 1 nW corresponds to:

$\text{P}_{\text{opt}} \; = \; 1 \times 10^{-9} \; \text{W = J/s} \; = \; n \, \mathcal{E}_\gamma \; = \; n \, \oldstylenums{128}e\text{-21}} \; \text{W = J/s} \; \; \Rightarrow \; \; \oldstylenums{7.81}e\text{9}} \; \gamma\text{/s}$

The basic photodiode is modelled as a reverse-biased semiconductor pn-junction. Semiconductor physics suggests that the balance of charge at a pn-junction forms a depletion zone – a region empty of free charge carriers – at the junction. The width of this depletion region increases as reverse-biased potential is increased. This forms a potential-dependent capacitance across the device. High frequency measurements tend to reverse-bias the diode at high voltages to reduce the shunt capacitance; that is not necessary for lower frequency measures such as those encountered with this project.

When a photon of sufficient energy strikes the lattice in the depletion region, it is absorbed into the structure by expending its energy into the release of an electron (a charge hole is also formed, but is not significant in this discussion). The electron is drawn to the device terminal; the movement of charge forms a current.

Noise in the photodiode primarily arises from one of two sources. A semiconductor junction is a delicately balanced structure of charge energy. Additional thermal energy can upset this balance and cause the release of an electron from the lattice. The aggregate of this energy transfer forms thermal current – commonly referred to as “white noise” which has a Gaussian probability distribution. The other primary noise source is created by the drift of charge carriers across the potential of a pn-junction. This is referred to as “shot noise”. This noise also has a Gaussian probability distribution.

“Flicker noise” is neither Gaussian nor stationary; it has a probability distribution inversely proportional to frequency and is sometimes referred to as “1 over f” noise. This is a low frequency phenomena; as the frequency of event increases, the magnitude decreases.

Thermal noise is often the most significant noise factor – this noise is reduced by a factor of the square root of the decrease in temperature. A reduction in temperature from “room” (300 K) to “LN77” (liquid nitrogen: 77 K) temperature will reduce theoretical thermal RMS noise by a factor of about ½.

$\frac{\text{Noise}_{77,rms}}{\, \text{Noise}_{300,rms} \, } \; \propto \; \sqrt{ \, \frac{77}{\, 300 \, } \, } \; = \, 0.5066$

This does not include additional noise sources related to cooling the detector. Shot and flicker noise are not temperature dependent (to 1st-order). The question to cool or not cool is deferred; this project assumes no cooling. In any case, it is more important to stabilize temperature than maintain a specific value; ΔT is more critical than T. Shot noise is usually not significant at moderate current levels but becomes more so at low signal magnitudes. Shot noise has a Poisson probability distribution with rms magnitude of 18 fA/rtHz at a DC current of 1 nA.

A noiseless photodiode has two primary parameters: dark current and responsivity. The dark current represents a “leakage” current within the structure – this current flows without optical stimulation. It has a Gaussian probability distribution consisting of both thermal and shot elements. Empirical measurements are more effective than analysis for determining dark current uncertainty. This dark current noise is usually less significant than other system noise sources but does establish the minimum detectable signal level.

To 1st-order, dark current is considered constant and represents the minimum device current. Optical stimulation adds to the dark current to form the device current such that the output is expressed as:

$\text{I}_{pd} \; = \; \text{I}_{dark} \, + \, \text{I}_{sig}$

In determining signal dynamic range, the dark current forms the lower signal bound. If the signal current is equal to the dark current, the dynamic range – at that signal magnitude – may be defined as 0 dB. This output current has 100% error.

Both dark and signal current flow in the same direction – this being a diode. The photodiode is reverse or zero biased; these currents are analogous to leakage current in a traditional diode. Electrical current is the movement of charge. Each electron carries a quanta of charge, 1.69E-19 coulomb; 1 C consists of 6.24E18 electrons.

The responsivity $\mathbb{R}$ in units of amperes/watt describes the relationship between optical power on the detector and the generated signal current. A responsivity value of 1 A/W is used in this discussion (the actual photodiode has $\mathbb{R}$ = 0.95 A/W). In terms of actual response, units of nA/nW are more realistic.

The system counts electrons by measuring charge for some defined period of time and reports the results. The number of electrons is related to the number of photons by the photodetector responsivity:

$\frac{\; \text{number of electrons} \;}{\text{time}} \; \; = \; \; \mathbb{R} \, \frac{\; \text{number of photons} \; }{\text{time}}$

Expanding:

$\mathbb{R} \; \equiv \; \frac{\; \text{amperes} \;}{\text{watt}} \; \; = \; \; \frac{\; \frac{ \; \text{coulombs} \; }{\text{sec}} \; }{\frac{ \; \text{energy} \; }{\text{sec}} } \; = \; \frac{\; \text{coulombs} \;}{\text{energy}} \; = \; \text{number of electrons} \;}{\text{number of photons}}$

The responsivity parameter defines the relationship between optical power and electrical current. Power and intensity have the same units if intensity is normalized to the detection area of the photodiode.

The selected photodetector has responsivity of 0.95 A/W

$\text{I}_{\text{pd}} \; = \; \frac{\, dq \, }{\text{dt}} \; = \; \text{P}_{\text{opt}} \, \mathbb{R} \; \; \Rightarrow \; \; 1 \times 10^{-9} \, \times \, 0.95 \; = \; 0.95 \; \text{nA}$

This corresponds to:

$7.81 \times 10^9 \; \gamma / \text{s} \; \; = \; \; 6.24 \times 10^9 \; \text{q/s}$

If a clocked system has frequency of 100 MHz, each incremental period converts:

$78.1 \; \gamma \; \; \equiv \; \; 62.4 \; \text{q}$

Charge
So perhaps considering the information as charge rather than current:

$\mathbb{R} \, \frac{\, \text{d} \gamma \, }{\text{dt}} \; = \; \frac{\, \text{dq} \, }{\text{dt}} \; \; \Rightarrow \; \; \frac{\, \text{d} \gamma \, }{\text{dt}} \; = \; \left. \frac{\, \text{dq} \, }{\text{dt}} \right|_{\mathbb{R}=1}$

While photodetectors may vary in material and wavelength response, they are all at heart simply diodes – pn-junctions – and once the photon-electron conversion has occurred, the signal from all types is simply a current. Common electronic processing procedures should be adaptable to a variety of photodiode types.

Although the match between number of photons and number of electrons is not quite 1:1, it is close enough to use in approximations such as for this discussion.

1 nW ( 1E-9 J/s ) of monochromatic optical energy will produce 1 nA ( 1E-9 C/s ) of current. Rather than relate watts to amps, consider number of photons to number of electrons. Monochromatic photons all have identical energy; electrons all carry identical charge.

The amount of charge accumulated in a given time is proportional to the number of impinging photons in that given time: the specific time period is not a factor. This allows time to be a controlling variable – integrate over time from 0 to T.

A capacitor is an electronic component which accumulates charge. As charge accumulates on the capacitor, the sum of the individual charges cause a voltage potential to develop across the capacitor.

Voltage and charge are related as:

$\text{Q} \; = \; \text{C V}$

A change in charge produces a change in voltage:

$\text{d}Q \; = \; C \, \text{d}V$

Related to time:

$text{d}Q \, }{\text{d}t} \; = \; C \, text{d}V \, }{\text{d}t}$

Noting that

$\frac{\, \text{d}q \, }{\text{d}t} \; = \; \text{I}$

Gives:

$\text{I} \; = \; C \, \frac{\, \text{d}v \, }{\text{d}t}$

Integrating:

$\int\limits_T I \, \text{d}t \; = \; \int\limits_T \frac{\, \text{d}q \,}{\text{d}t} \, \text{d}t \; = \; C \, \int\limits_T \frac{\, \text{d}v \,}{\text{d}t} \, \text{d}t$

$\int\limits_T I \, \text{d}t \; = \; \text{Q} \; = \; \text{C V}$

A rather roundabout way of stating that by integrating the photodiode current over some time period T, the accumulated charge – directly related to optical intensity – may be inferred from the voltage developed across a known capacitance. A simple switch is used to “reset” the capacitor after time T.

That’s good for now.

$^\textbf{\normalsize 1}$ The mechanics of generating the laser beam and the physics of the photon-to-electron transformation in the photodiode are of interest … at that level. For the purposes of extracting the desired information, the photodetector produces a current having a linear relationship to beam intensity. Ideally, 1 photon produces 1 electron. All else is scaling.

$^\textbf{\normalsize 2}$ Using “more realistic” approximations, H = 10 km,  = 0.17 mrad  TX volume = 30,264 m3
With an 8” receive lens, the RX volume is 30,443 m3. The RX volume is 0.6% larger than the TX volume. If identical paths are assumed, the ideal geometry error exceeds the required measurement precision. What this implies of course is that the absolute error in concentration is far more sensitive to the uncertainty in measurement volume than uncertainties in the electronic chain. This has more effect on the dynamic range of the system than the differential system … within the suitable common-mode rejection range.